When you look up in the sky and you see a contrail, how far away is it? How far away are these contrails, a mile? two miles? Would you believe they are actually 20 to 100 miles away?

Contrails typically form above 30,000 feet, or around six to eight miles straight up. It’s quite hard to judge exactly how high it is unless you know what type of plane it is. But assuming six miles is a pretty safe bet.
So if we assume it’s at least six miles above the ground, then all we need to do to find out how far away it is is to measure the angle of elevation. It’s quite a common child’s math problem but usually applied the other way around, to measure the height of things if we know how far away they are.
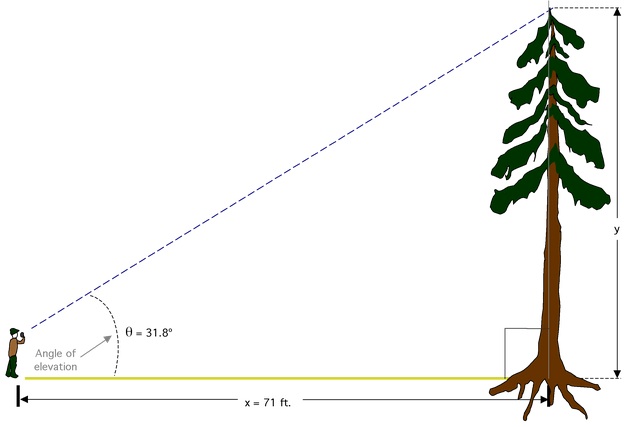
To measure the height …
So it’s a really simple bit of maths, height = distance * tan(angle).
But of course, if you know the height, then you can calculate the distance = height * (1 / tan(angle)).
Now most planes leaving contrails cruise at between 30,000 and 45,000. 6 miles is 31,680 feet, so it’s a pretty safe assumption that any contrail you see is at 6 miles or above. So for the purposes of calculating the distance, let’s just assume it’s six miles high. At worst we will underestimate. Here’s what the figures work out as for various angles from the horizon:
| Angle | 1/TAN(Angle) | Height | Miles | LOS | Sq Miles | km | Sq Km |
| 5 | 11.4 | 6 | 68.6 | 68.8 | 14776 | 110.4 | 38269 |
| 10 | 5.7 | 6 | 34.0 | 34.6 | 3638 | 54.8 | 9421 |
| 15 | 3.7 | 6 | 22.4 | 23.2 | 1575 | 36.0 | 4080 |
| 20 | 2.7 | 6 | 16.5 | 17.5 | 854 | 26.5 | 2211 |
| 25 | 2.1 | 6 | 12.9 | 14.2 | 520 | 20.7 | 1347 |
| 30 | 1.7 | 6 | 10.4 | 12.0 | 339 | 16.7 | 879 |
| 35 | 1.4 | 6 | 8.6 | 10.5 | 231 | 13.8 | 597 |
| 40 | 1.2 | 6 | 7.2 | 9.3 | 161 | 11.5 | 416 |
| 45 | 1.0 | 6 | 6.0 | 8.5 | 113 | 9.7 | 293 |
| 50 | 0.8 | 6 | 5.0 | 7.8 | 80 | 8.1 | 206 |
| 55 | 0.7 | 6 | 4.2 | 7.3 | 55 | 6.8 | 144 |
| 60 | 0.6 | 6 | 3.5 | 6.9 | 38 | 5.6 | 98 |
| 65 | 0.5 | 6 | 2.8 | 6.6 | 25 | 4.5 | 64 |
| 70 | 0.4 | 6 | 2.2 | 6.4 | 15 | 3.5 | 39 |
| 75 | 0.3 | 6 | 1.6 | 6.2 | 8 | 2.6 | 21 |
| 80 | 0.2 | 6 | 1.1 | 6.1 | 4 | 1.7 | 9 |
| 85 | 0.1 | 6 | 0.5 | 6.0 | 1 | 0.8 | 2 |
| 90 | 0.0 | 6 | 0.0 | 6.0 | 0 | 0 | 0 |
The important column there is in bold, the “Miles” column which tells you how far away horizontally the plane is. That is, it tells you how far away the point is that is on the ground directly below the plane (ignoring curvature of the earth, and local changes in height).
The column next to that, LOS (Line Of Sight) tells you the actual distance from you directly to the plane. So when it’s overhead it’s 6 miles (straight up). As it gets further away it gets closer to the horizontal distance.
Look at the angles below 45 degrees. In particular, a plane that’s ten degrees above the horizon will be 34 miles (55 km) away, and one that is five degrees will be 68 miles (110 km) away. Now ten degrees might not seem like a lot but it actually is surprisingly high if you go out and point your arm up at ten degrees.

Here I’m pointing up at ten degrees, I see plenty of planes “over there”, it seems pretty unintuitive to think that they are 50 miles away. But they are. Notice (as you would expect) at 45 degrees, the plane is the same distance away as the height, six miles. And any angle above 45 degrees is within a six-mile radius. But still, consider that many people will point to a plane at 45 degrees as being “overhead” when really it’s six miles away.
Now let’s look at some actual contrails. These photos were taken on an iPhone using the “Theodolite” app, which tells you the angle of elevation.
Contrails at five degrees, so around 70 miles away
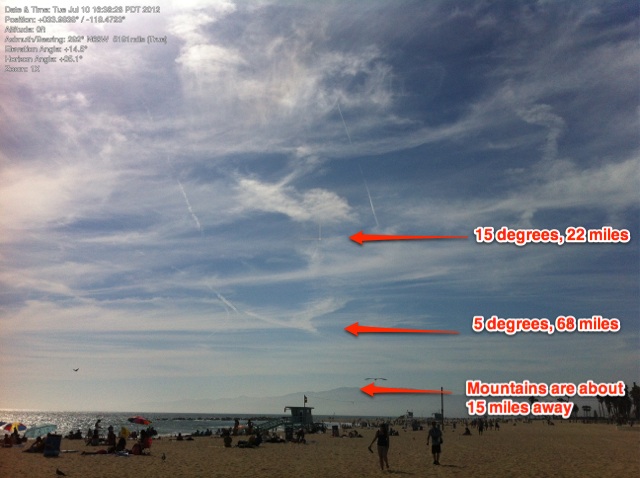
Contrails are generally at the same altitude. So if a contrail is “below” another, that means it’s further away. In this case 46 miles further away. Notice how much further away the contrails are than the mountains, but they seem visually closer.

Here you might describe this contrail as “flying right over the yellow house”, but at 20 degrees elevation, it’s AT LEAST 16 miles away – way out away from the city.

You can also use this to get an estimate of the length of a contrail segment if it’s coming towards you. Here we can calculate the contrail goes from 12 to 27 degrees, so is about 16 miles long. It looks like it’s vertical, but it’s actually horizontal.
This math breaks down somewhat for below five degrees, as they the curvature of the earth is much more of a factor. But for most contrails, you see you can get a reasonable estimate of the distance from the angle. And since most contrails do not fly directly overhead, the vast majority of visible contrails are going be at a low angle, and very far away. Much further than you might think.
Back to the photo we started with. It was taken from a bridge over the Thames to the west of London. Using Google Earth, I’ve placed a grid at six miles altitude, with five miles between each line.

Contrails, looking west from Kew Bridge over the Thames, to the West of London. It looks like the contrails are “over the city” but really they are much further away
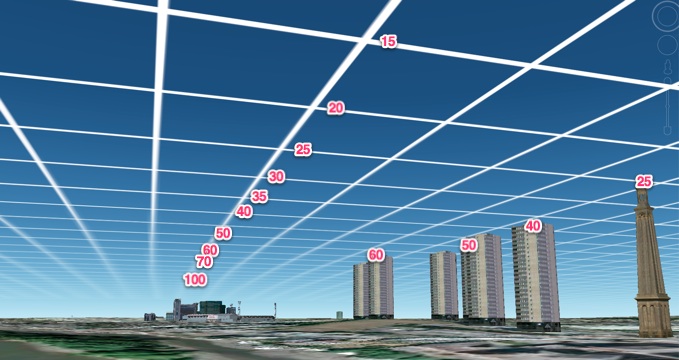
Drawing a grid at six miles up shows just how far away. The closest would be ten miles away, the furthest over 100 miles. The number at the top of the buildings don’t show the distance to the building (actually less than a mile), but show the distance to something six miles up in the sky if it appeared directly behind the top of the building.
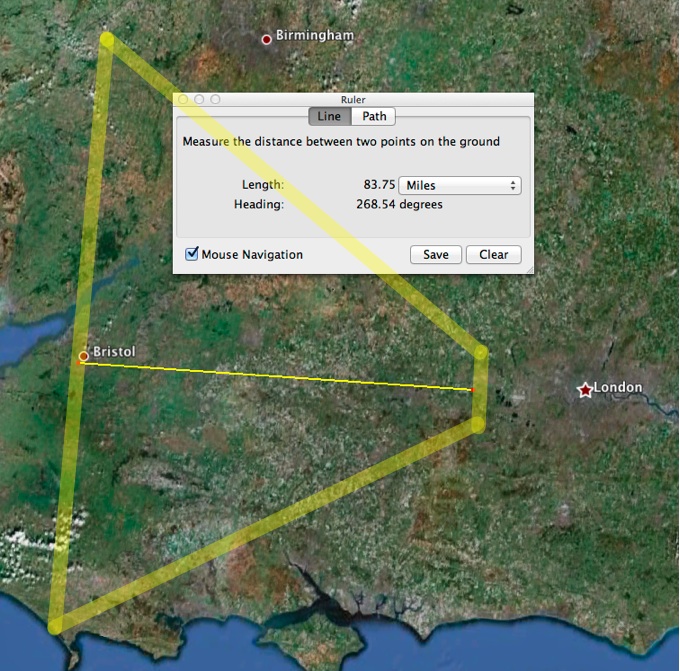
If we draw out the visible cone on a map, we see that the contrails are mostly far out over the countryside, nowhere near the city at all. Most likely they are intercontinental flights from Continental Europe to North America

Thanks for this article.
But could you be so kind to add metric distances to the table for thew non-american readers?
Done!
It would be useful to have line of sight distance too.
I’ve added line of sight distances. For the contrails close to the horizon it’s pretty close to the horizontal distance. LOS is useful if you are calculating the size of the plane, or using that as a variable in some other calculation.
I had an astronomy class last year and they taught us a method for finding the distance of something when you do not know the height…it involved using your outstretched arm held in front of your face, with your thumb up, and you used an object close to you in which you DO know the distance to compare and determine the distance of a far away object…I can not remember much more about this method, but its what astronomers use to find how far away a star system is, or a planet.
That can’t possibly be right. Astronomers may use that method but it’s not with their thumbs.
I guess I should say this a different way, its how astronomers used to find the distance of something in space, before they had telescopes. Kind of a way of ancient experiments.The way I put it in the previous post made it sound like they still do it that way lol.
The outstretched arm, thumb up, measure the proportion of the moon’s width against the profile of your thumb.
The Moon: Distance 220,000 mi 2000 mi in diameter Ratio 100:1 Ballpark
Aircraft 100 ft long appears to match portion of thumb tip covered by moon: aircraft is 10,000 ft away. 2 mi
Ballpark
Thanx for your page , been doing this for years, no validation on ‘net until I typed in distance to “Contrails”!
Getting the moon in shot would actually be a great way of measuring the distance to the plane, as it’s more or less fixed in size (about 10% variance I think). It does depend on being able to identify the plane though.